기계진동학에서 문제를 풀다보면 보통 미분방정식 형태로 많이 나온다. 이 미분방정식은 그냥 풀 수 있는 건 아니고 boundary condition이 주어져야 풀 수 있다.
대표적인 boundary condition
1) Dirichlet boundary condition
boundary condition 중에 종속 변수의 0차 미분에 대한 condition이다.
예시를 보면서 이해해보자.
Example)
for
이 방정식은 1차원 2차 편미분 방정식인 라플라스 방정식이므로 initial condition 2개, boundary condition 2개가 필요하다.
다음과 같은 라플라스 방정식에서 조건을 다음과 같이 줄 수 있다.
지금 보면, t에 관계 없이 공간에 대한 boundary condition으로 일 때 종속변수 에 대한 condition을 주었다.
2) Neumann boundary condition
boundary condition 중에 종속 변수의 1차 미분에 대한 condition이다.
Example)
다음과 같은 2차원 heat equation의 경우
for
x와 y에 대해 각각 2개의 boundary condition이 필요하다.
이 때
다음과 같이 x와 y에 대한 1차 미분에 대해서 2개씩 boundary condition을 가진다.
3) Robin boundary condition
Robin boundary condition은 Neumann condition의 일반화된 버전이다.
앞에 붙은 은 상수이다. 이 상수를 보면 왜 이 조건이 Neumann의 일반화 버전인지 알 수 있다.
이면 사실 상 Neumann boundary condition과 형태가 동일해지기 때문이다.
위의 condition은 diffusion 또는 convection equation에서 자주 쓰인다고 한다.
Condition 예시 - Free vibration of shaft
기계공학에서 나오는 대표적인 미분방정식 중 하나인 free vibration of shaft을 보면서 위의 condition이 적용된 예시를 본다.
위 방정식에서 x는 공간에 대한 변수, t는 시간에 대한 변수이고 는 변위를 의미한다.
이 미분방정식을 풀기 위해서는 몇 개의 condition이 필요할까?
시간에 대한 2차 미분이므로, initial condition 2개, boundary condition이 2개가 필요하다.
이 때 구속조건은 다음과 같이 주어질 수 있다.

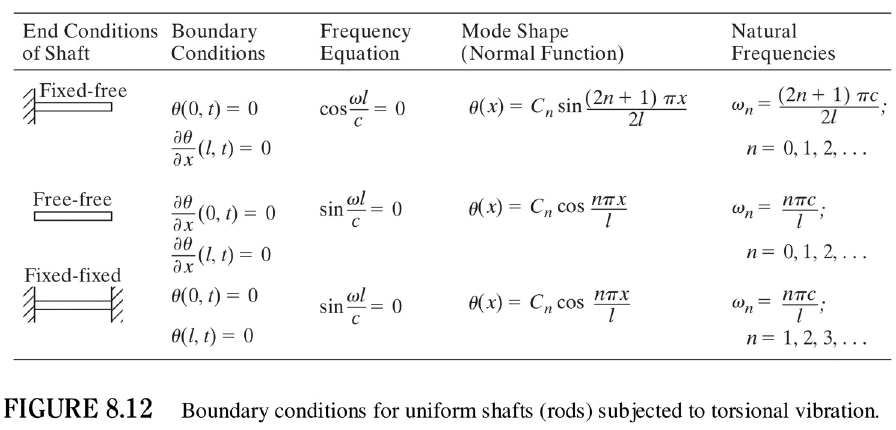
Rao의 mechanical vibration 책을 보면 다음과 같은 그림을 찾을 수 있다.
맨 위처럼 구속조건이 있는 경우는 0차 미분, 1차 미분 둘 다 있는 경우이다.
두 번째로 양 끝이 free인 경우에는 Neumann boundary condition이 적용된다.
마지막으로는 양 끝이 고정된 경우에는 Dirichlet boundary condition이 적용된다.
boundary condition 중에 Cauchy boundary condition도 있고 더 주어져있다.
궁금하면
https://en.wikipedia.org/wiki/Category:Boundary_conditions
Category:Boundary conditions - Wikipedia
en.wikipedia.org
이 쪽으로 들어간다.
아래는 Cauchy boundary condition이다. 1차 미분과 0차 미분 모두 포함하는 경우를 의미한다.

'수학 Mathematics > 미분방정식 Differential Equation' 카테고리의 다른 글
| [Differential Equation] 시작 (0) | 2023.12.12 |
|---|---|
| [미분방정식] 미분방정식 답을 간단하게 알고 싶을 때 사용하는 방법 (0) | 2022.04.07 |